
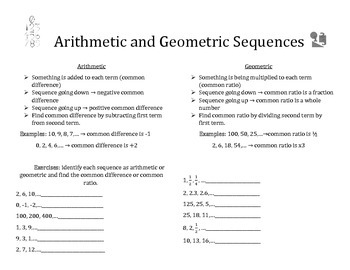
For example: 1, 4, 7, 10, 13, 16, 19, 22, 25, This sequence has a difference of 3 between each number. The differences between the two sequence types depend on whether they are arithmetic or geometric in nature. We just add some value each time on to infinity. Arithmetic Sequence In an Arithmetic Sequence the difference between one term and the next term is a constant. Arithmetic sequences consist of consecutive terms with a constant difference, whereas geometric sequences consist of consecutive terms in a constant ratio. The primary focus will be on arithmetic and geometric sequences. The differences between the two sequence types depend on whether they are arithmetic or geometric in nature. To this end, an Arithmetic and Geometric approach are integral to such a calculation, being two sure methods of producing pattern-following sequences and demonstrating how patterns come to work. The patient is told to walk a distance of 5 km the first week, 8 km the. A recovering heart attack patient is told to get on a regular walking program. A sequence that involves multiplying (or dividing) by the same value each time. The terms consist of an ordered group of numbers or events that, being presented in a definite order, produce a sequence. A sequence that involves adding (or subtracting) the same value each time. Using the formula for the general term, a n11 a n 5 2n11 2n 5 2. There are decimal and fractions included in the terms.There are two versions of. The sequences are all numeric and there are none with formulas. Use the "Calculate" button to produce the results. (b) a 2 2 a 1 5 4 2 2 5 2, and a 3 2 a 2 5 8 2 4 5 4, so the sequence is not arithmetic. This Arithmetic and Geometric Sequences sort is a great interactive activity for your students to differentiate between sequences which are Arithmetic, Geometric, or Neither.Insert common difference / common ratio value.Insert the n-th term value of the sequence (first or any other).Use the dropdown menu to choose the sequence you require.then you know that you have to add 3 to get the next number. For instance, if you have a sequence, 2, 5, 8, 11, 14. The arithmetic sequence works when you have to add or subtract the same value to get the next number in the sequence.

As you can see, every term is actually just a different power of 3:ģ 0, 3 1, 3 2, 3 3, 3 4, 3 5, … Who wants to be a Millionaire?Īby odsłonić więcej treści, musisz wykonać wszystkie powyższe czynności i ćwiczenia.By applying this calculator for Arithmetic & Geometric Sequences, the n-th term and the sum of the first n terms in a sequence can be accurately obtained. However, two of the sequences which are the easiest to solve are the arithmetic and geometric sequence. This sequence of numbers has a special name: the powers of 3.

Show the class some examples of objects or pictures that have a repeated pattern. It was a comet, a small, icy rock that is flying through space, while leaving behind a trail of dust and ice. In the 10th step, you would reach 19,683 new ones, and after 22 steps you would have reached more people than are currently alive on Earth. geometric sequence, arithmetic sequence, common ratio, common difference (7.2) Student/Teacher Actions (what students and teachers should be doing to facilitate learning) 1. Arithmetic and Geometric Sequences In 1682, the astronomer Edmond Halley observed an unusual phenomenon: a glowing white object with a long tail that moved across the night sky. The number of people increases incredibly quickly. Using the explicit formula for geometric sequences, we can work out how many new people are affected at any step: ID: 1 Secondary I Name 4. If we want to find any term (as the n term) in the arithmetic sequence, we can. An arithmetic sequence is a list of numbers with a defined pattern. Notice how the number of people at every step forms a geometric sequence arithmetic sequence triangle number, with common ratio : View Arithmetic and Geometric Sequences Worksheet.docx from MATH 105-1-1 at Spectrum High School. Arithmetic and Geometric Sequences Formulas and Examples Arithmetic sequences. The essence of Trevor’s idea is that, if everyone “pays it forward”, a single person can have a huge impact on the world: Arithmetic -1- ©3 a2F0h1 720 DKvuDt TaS fS Bo GfftBw BadrIe m WLBLPC m.f 7 yA wl 7lR yrLifgYh 2tPs L WrveIs Jeqr1vhe AdV. This video was built as part of the learning resources provided by the Western Canadian Learning Network (a non-profit collaboration). Extract from “Pay It Forward” (2000), © Warner Bros.


 0 kommentar(er)
0 kommentar(er)
